MULTIMAGIE.COM - February 2017 news

List of the site's news added February 14, 2017 (and February 24)

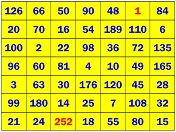
Solution to enigma #6b! By the same Frenchman, a year and a half after his solution to enigma #4c.

First known 7x7 add-mult magic square, by Sébastien Miquel, winning again €200, and again a bottle of champagne, happy man!
This square becoming the smallest known add-mult magic square, smaller than the previous records (W. Horner's 8x8 and C. Boyer's 8x8), updated table of known add-mult squares

With this new enigma solved, there remain eight unsolved enigmas for winning €6,500 and eight bottles of champagne!
All the enigmas on 7x7 squares having been now solved, the remaining enigmas are on small squares, from 3x3 to 6x6. Here are the most interesting recent results on them:

#1 (3x3 magic square of squares, or using at least 7 distinct squared integers), new searches from Terry Moriarty, Eddie Gutierrez, Matt Parker (with Brady Haran), Ben Asselstine.
Fun video with Matt Parker trying to solve the enigma #1.
#3 (3x3 semi-magic square of cubes), no solution using taxicab numbers ≤ 2*10^13found by Tim Roberts, using one of Morgenstern's methods
#6 (5x5 add-mult magic square), interesting add-mult semi-magic squares by Keith Lynch

Bimagic square of order 9 with numbers of nine digits using all digits from 1 to 9 constructed by Mitsutoshi Nakamura and Inder Taneja
Pandiagonal bimagic square of order 385 constructed by Li Wen, difficult to construct such squares of order pqr, here 385 = 5*7*11
Various bimagic construction methods compiled by Holger Danielsson, in a PDF of more than 100 pages! (in German)

New best known 16x16 pandiagonal multiplicative magic square, by Oscar Lanzi, leading to an updated table and Excel file of best known pandiagonal multiplicative magic squares
60^6 is the smallest possible product of 6x6 pandiagonal multiplicative magic squares, proof proposed by Oscar Lanzi

Perfect bimagic cubes of orders 16 and 25 constructed by Zhong Ming; they are the new smallest known perfect bimagic cubes, smaller than my perfect bimagic cube of order 32

Second edition of "Latin Squares and their Applications" now available (Elsevier 2015).
This famous book by Donald Keedwell and Jószef Dénes, foreword by Paul Erdös, first published in 1974, is revised and gives the present state of knowledge on Latin squares.
Many thanks to Donald Keedwell for several references to my work and to this website!
Good news on Frost's cube of 11, and new photo by John Williamson, added in Frost's biography. This cube has now been conserved and is in excellent condition in the Holloway College archives.
Updated biography of Georges Pfeffermann (in French, but summary in English) on his last years in Issoire, thanks to Gildas Guillemot who had the idea to search in the archives of Le Moniteur d'Issoire.
Inder Taneja's webpages added in the links

This retired mathematician living in Los Angeles, California, can no more be reached through his email address at earthlink.net ("550 User account is unavailable"). My last exchange of messages with him was in May 2015. His webpages http://home.earthlink.net/~morgenstern are no longer available ("403 Forbidden") since at least January 2016, and were unfortunately not fully saved by the Wayback Machine.
~~If you have any info on this author of numerous results reported in multimagie.com since 2006,~~ ~~send me a message~~!

< Morgenstern's old webpages at earthlink.net (click to enlarge)

Added February 24, 2017

I succeeded in contacting Lee Morgenstern, and good news, he is well!
Because his webpages are no longer available, his interesting counting method was "lost", with a dead link from my page on multimagic series.
He kindly sent me a PDF: bimagic series counting method, by Morgenstern in 2013, now again available.